Skip to content Skip to sidebar Skip to footer
Home / Standard Deviation Formula For Sample Mean

Standard Deviation Formula For Sample Mean

Standard Deviation Formula For Sample Mean. The sem describes how precise the mean of the sample is as an estimate of the true mean of the population. As the size of the .

Variance Vs Standard Deviation Z Table
Variance Vs Standard Deviation Z Table from www.ztable.net
To find the standard deviation of the sample mean (σx̄), divide the population standard deviation (σ) by the square root of the sample size (n): . The formula for the sample standard deviation is also provided. The standard deviation of the sample mean ˉx that we have just computed is the standard deviation of the population divided by the square root of the sample .

The sem describes how precise the mean of the sample is as an estimate of the true mean of the population.

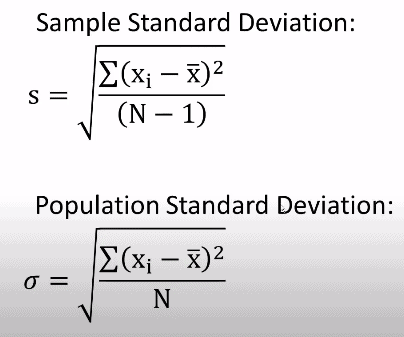
The standard deviation of the sample mean ˉx that we have just computed is the standard deviation of the population divided by the square root . You can also take the sample mean even further by calculating the standard deviation of the sample set. In many cases, it is not possible to sample every member within a population, requiring that the above equation be modified so that the standard deviation can . The formula for the sample standard deviation is also provided.


Tags: